fractals

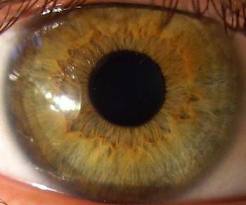
Every day we see patterns and understand that someone lot of trouble to create them. And what about the patterns found in nature? What are they hiding?
For example, take a snowflake. These crystals form when water vapor in the cloud is transformed into ice. With the increase in the size of the crystals appear delicate openwork patterns. If we consider a single snowflake, we can see that its rays branch again and again, forming the rays of smaller dimensions. This property is self-similarity of mathematics called fractal. Fractal - a kind of figure in which one and the same motif in the Carbonless gradually decreasing scale.
The concept of fractal geometry and fractal, born at the end of the 70's, from the mid 80's overhaul settled and become current programmers and mathematicians. The word fractal is of Latin origin and is translated from fractus as consisting of fragments. It was proposed in 1975 by Benoit Mandelbrot to describe irregular structures, but still self-similar, which he carried away.
Fractals - great education, unpredictable movements generated by the chaos of the world. They are observed in such tiny objects, like the cell membrane, and such extensive, like the solar system.
If we consider a sea shell, you'll find that it is a fractal structure in the form of self-similar compartments, which increases during the life of the mollusk.
Cabbage, cauliflower, broccoli, pineapple, sunflower seeds are also fractals, as Use the same principle of self-similarity and nesting.
The famous toy "Matryoshka" - a real model fractal. Fractal principle obvious, when toy figurines of wood arranged in a row, and not nested within each other out.
Fractals can be expressed in furniture design. For example fractal nightstand from Japanese designer Takeshi Miyakawa uses maximum storage space and looks very stylish.
Fractals are increasing and significant application in science. The main reason for this can be called what they describe the world around us, sometimes better than a traditional physics or mathematics. Here are just some of the areas of application: computer graphics, physics, radio engineering, economics, and biology. Besides all the practical, fractals just give aesthetic pleasure

What is a gyro?

Gyro called rotating body (for example, a massive disk), which is set in a fairly rapid rotation around some axis of symmetry. Initial acquaintance with a gyroscope usually occurs in childhood when observing the famous toy - baby peg or yuloy.
Until the top is rotated rapidly, it can be kept stable on its axis and keep upright. He does not fall on a horizontal plane, despite the fact that the center of gravity is higher than the pivot. This is possible because of the conservation of angular momentum. In the case of deviation of the axis of a spinning top from a vertical direction by gravity axis in space describes a particular circular cone, so the axial tilt angle does not change. Such a rotation of the gyroscope is called a forced precession.
Precession watch very simple. You should start a top and wait until it starts to slow its rotation. The top point is beginning to fall and the spiral (divergent). This is called the precession of the axis.
Slight irregular motion of rotating rigid body, which makes the precession is called nutation. Such movement is somewhat reminiscent of "twitch" axis of rotation.
Consider some uses gyro.
Features gyroscope are widely used in helicopters to stabilize their balance. As a rule, the helicopter has a 2.3 gyroscope to stabilize the horizontal and the vertical. Ie different variations of the helicopter and vibration muffled by a gyroscope, which were caused by its powerful engines and propellers;
stabilization in the horizontal border speedboats. With the development of high-speed boats of big waves on the water surface run powerful gyroscope, which smooths out the pounding waves on the bottom of the boat.
gyroscopic instruments are of great use to automate the management of ships and aircraft. To support the set of a ship includes autopilot and the aircraft - the autopilot;
demanded gyroscopes in automotive safety and comfort, to control input and stabilize the camcorder. Gyroscopes are used to adjust the height and stability of the suspension.

Possibility of the impossible: travel to the past and the future.

The ability to move in time to look into the future and change the past, or simply to experience pleasant moments again, centuries worried inquisitive mind of man. But today, scientists are in place so far all attempts to find a reasonable answer to the actual question of how you can teleport in so unstudied substance as time, relying only on the claim that there is no proof you can not move in it. Albert Einstein mathematically proved that time travel is possible when the object faster than the speed of light (3 * 108 m / s).
A black hole, according to science, has enormous power of attraction, and, on this basis, while near its center runs very slowly. That is all you need to travel in time - this is just a temporary move from the field of the Earth at the time of the black hole in a matter of seconds. An alternative way of moving through time and space - the use of so-called wormholes. According to quantum physics, it is possible to move into the past or future, to jump from the Earth (or any other entry points) to infinity galaxy or universe. The problem is that the wormhole is too small for the passage of people, so the first task of this generation will be the science of energy, which will expand the walls of the hole. Further, it is impossible to get "much anyhow": the "one" side, you should see the arrival, and at the same portal should be kept open. We conclude that the return to the past, which does not have this very point of arrival, it becomes impossible. Modern scientists have "fun" trying to send a photon in the past, and then change its polarization.
Recently formulated a theory was the idea of ​​Bella that all points of space have instant communication, but to be able to transfer it to the subject does not have the energy. In other words, to move in the blink of an eye can still only information.
So how do scientists need to contrive to finally get to another time, when you want? Of the most common theories of the conclusion is the following: either learn a speed greater than the speed of light, or to reproduce the energy is so strong that it can push the gravity field, opening the gates to other times and universes.

Another mystery of nature

Reproaches toward chemists is that they create a dangerous and "unnatural" substances are accompanied by such adjectives as "toxic", "toxic" pollutant "," explosive ", etc. This is happening at a time when organic chemistry can create perfection.
Modern humanity is unlikely to yourself without thinking billion of various products of organic synthesis. These are synthetic fibers, drugs, and more, are made on the plants of the chemical industry.
Proud application of modern organic chemistry synthesis capabilities of both known and unknown nature of the compounds immediately fade when compared with the abilities of nature.
For example consider a living cell - an "atom of life." Enzyme catalysts in it thousands of compounds synthesized in a very modest initial conditions - no heat, pressure, halogens, strong acids, bases and other "frills." In the cell, not just the biological molecules are synthesized, but also fine microstructure formed in insane amounts. These microscopic entities biologists gave the name "organelles" (from the Greek. «Organan», which means "an instrument, a tool, a body"). The cell secretory organelles perform the receptor, motor, and other functions. Interestingly, the organelles are sverhmolekulyarnye structure.
Organic compounds in nature occur with tangible quantitative yields in minutes! This suggests that the tactics and strategy of fusion (which chemists have so far only dream) has long been solved by nature and function successfully in the "chemical plants" of any living system.
Consider an ordinary green leaf. Foliage nature annual "stamp" in incredible amounts. But you can handle this task in the laboratory modern organic chemist using the latest equipment and research and development? The answer - no!
Nature hides another secret. It is not only able to create super complex biomolecules, but cleverly and intricately combine them to form the intricate structure, which she deftly manipulates.
As nature has learned it needs to create molecular formations and manage them? This is another mystery of nature, which will reveal.

Scientists have managed to create a retinal prosthesis, which does not require a power source

Scientists have developed a new prototype electronic retina, suitable for high-definition images and does not require external power.
U.S. biotechnologists created a prototype artificial retina of the human eye, which does not require a power supply and operating at infrared energy, as described in an article in Nature Photonics.
Currently in the world, scientists are developing a number of different types of implants that are theoretically able to return vision lost due to accidents or degenerative diseases. Biologists experiment, in some cases, with individual retinal cells and stem cells, in others - biotechnology and physical and try to combine the work of a variety of electronic devices with the work of the brain of animals and humans. But until now, no study has been able to achieve significant success.
A team of scientists at Stanford University in the U.S. under the direction of James Loudin was able to develop a new prototype electronic retina, which is suitable for high-definition images and does not require an external power supply, which is the main obstacle to the development of such technologies.
"Made us invention operates approximately the same principle as the solar panels installed on the roof of the house - converts light energy into electrical impulses. However, in this case electricity supplies are not "TV" and sent directly to the retina as a signal, "- explained one of the band members Daniel Palanker.
New artificial retina created James Loudin and his colleagues, as a set of a large number of individual microscopic silicon plates, which combine the power generator, a light sensor, and some other items. To this retina work, you must have the special glasses with built-in video camera which, and a small pocket computer, which processes the image.
This invention works as follows: being in glasses camera continuously converts the light rays into electronic pulses. Each "frame" is then processed by a computer and is divided into two halves - the left and right eyes, and the information is sent to the infrared heaters that are on the inside of the eyeglass lens. Points then emit infrared radiation in the form of short pulses that activate photocells located on the retina. Infrared radiation causes photosensors transmit electrical impulses encodes pictures in optical neurons.
"Today implants very cumbersome operation to insert into the eye of all the necessary components are incredibly complex. Now, in our case, the surgeon is enough to make the retina is only one small incision and immerse photosensitive component of the device under it "- says Palanker